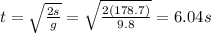Question

A 3.97 kg ball is dropped from the roof of a building 178.7 m high. While the ball is falling to Earth, a horizontal wind exerts a constant force of 12.3 N on the ball. How long does it take to hit the ground? The acceleration of gravity is 9.81 m/s² . Answer in units of s.

Answer 1

6.04 s

Step-by-step explanation:

The time of flight of the ball is not influenced by forces acting along the horizontal direction, but only on the forces acting on the vertical direction: this means that we can totally ignore what happens along the horizontal direction (where there is the force of the wind acting) and we can focus only on the vertical motion of the ball, which is only influenced by gravity.

Therefore, since the vertical motion of the ball is a uniformly accelerated motion, we can use the following suvat equation:

`s=ut + (1)/(2)at^2`

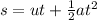
where, choosing downward as positive direction, we have:

u = 0 is the initial vertical velocity

s = 178.7 m is the vertical displacement

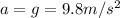`a=g=9.8 m/s^2` is the acceleration of gravity

t is the time

Solving the equation for t, we find after how much time the ball hits the ground:

`t=\sqrt{(2s)/(g)}=\sqrt{(2(178.7))/(9.8)}=6.04 s`