Question

Consider the function f(x)=
`√(x+2)-6` for the domain [-2,∞)

Find
`f^(-1)(x)`, where
`f^(-1)` is the inverse of
`f`.
Also state the domain of
`f^(-1)` in interval notation.

Answer 1

• f^-1(x) = (x+6)^2 -2
• domain: [-6, ∞)

Explanation:

To find the inverse function of f(x), we solve for y the equation ...

x = f(y)

x = √(y+2) -6

x +6 = √(y +2)

(x +6)^2 = y + 2

(x +6)^2 -2 = y

The inverse function is ...

`f^(-1)(x)=(x+6)^2-2`

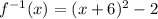
The domain of f^-1 is the range of the function f(x): [-6, ∞).