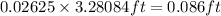Question

A passive solar home has energy stored in a concrete floor of 1000 square feet. How thick should this floor be to store 150000 Btu with a temperature swing of 20 degrees F? (Compute the answer in feet. If the floor should be one tenth of a foot thick, enter 0.1) (Note: you'll need to find a value in your textbook for the energy storage capacity of concrete)

Answer 1

Thickness is 0.086 ft

Solution:

iAs per the question:

Temperature, T =
`20^(\circ)F = 36^(\circ)C`

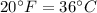
Energy to be stored, E = 150000 Btu

Area of the concrete floor, A =
`1000\ ft^(2) = 93 m^(2)`

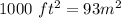
Density of concrete,
`\rho = 2400\ kg/m^(3)`

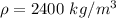
The heat energy is given by:

`Q = ms\Delta T` (1)

Also, we know that:

1 Btu = 1055 J

`\rho = (m)/(V)`

`m = \rho V`

`V = A* t`

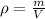
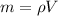
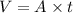
where

`\rho` = density

m = Mass

V = Volume

A = Area

t = thickness

s = 750 Jk/kg

Now, eqn (1) can be written as:

`Q = \rho * A* t* s\Delta T`

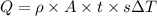
Thickness can be written as:

`t = {Q}{\rho * A* s\Delta T}`

`t = {150000* 1055}{2400 * 1000* 0.093* 750* 20* ((9)/(5))}`

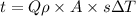
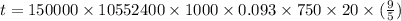
t = 0.02625 m

t =
`0.02625* 3.28084 ft = 0.086 ft`