Answer:
a) 25
b) 49
c) 97
Explanation:
The sample size is calculated using the formula, n =
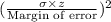
Now,
for 95% confidence level value of z-factor = 1.96
Given:
Mean = $3.94
standard deviation = $0.25
thus,
a) for margin of error = $0.10
n =
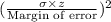
or
n =

or
n = 4.9²
or
n = 24.01 ≈ 25 (Rounded off to next integer)
b) for margin of error = $0.07
n =
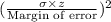
or
n =
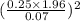
or
n = 7²
or
n = 49
c) for margin of error = $0.05
n =
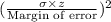
or
n =

or
n = 9.8²
or
n = 96.04 ≈ 97 (Rounded off to next integer)