Answer:
y = 428.67 m and x all = 1513.68 m
Step-by-step explanation:
This problem of kinematics can be divided into two parts: a first part when the rockets work a second as a parabolic launch.
Let's do the first part, let's calculate the speed just when the engines turn off
vf = v₀ + at
vf = 78 + at
vf = 78 +12 3
vf = 114 m / s
This is the speed with which the second part begins vo = 114 m / s with an Angle of 38º
Also at this time a distance is displaced, we calculate the distance traveled (in the direction of the acceleration)
d = v₀ t + ½ a t²
d = 78 3 + ½ 12 3²
d = 288 m
Let's use trigonometry to find the components
x₀ = d cos 38 = 288 cos 38
y₀ = d sin38 = 288 sin38
x₀ = 226.95 m
y₀ = 177.31 m
Second part
Let's calculate the maximum height, at this point its vertical speed is zero (vfy = 0)
Let's decompose the initial velocity using trigonometry
vₓ = v₀ cos 38
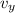 = v₀ sin38
= v₀ sin38
vₓ = 114 cos 38
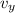 = 114 sin38
= 114 sin38
vₓ = 89.83 m / s
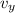 = 70.19 m / s
= 70.19 m / s
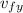 ² =
² =
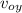 ² - 2g (y -y₀)
² - 2g (y -y₀)
0 =
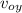 ² -2g (y -yo)
² -2g (y -yo)
y-y₀ =
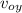 ² / 2g
² / 2g
y-y₀ = 70.19²/2 9.8
y = 251.36 + y₀
y = 251.36 + 177.31
y = 428.67 m
This is the maximum height from the point where the movement began, that is, the ground.
Now let's calculate the range
R = vo² sin 2θ / g
R = 114² sin 2 38 /9.8
R = 1286.73 m
This is the scope of the parabolic movement, we must add the horizontal distance traveled in the first part
x all = R + xo
x all = 1286.73 + 226.95
x all = 1513.68 m