Question

There was no snow on the ground when it started falling at midnight at a constant rate of 1.5 inches per hour. At

4:00 a.m., it starting falling at a constant rate of 3 inches per hour, and then from 7:00 a.m. to 9:00 a.m., snow was
falling at a constant rate of 2 inches per hour. It stopped snowing at 9:00 a.m. (Note: This problem models snow
falling by a constant rate during each time period. In reality, the snowfall rate might be very close to constant but is
unlikely to be perfectly uniform throughout any given time period.)
d. How deep was the snow at 9:00 a.m.?

Answer 1

The depth of snow at 9:00 am was 19 inches.

Explanation:

Consider the provided information.

Let x represents the number of hours and S(x) represents the depth of snow.

There was no snow on the ground when it started falling at midnight at a constant rate of 1.5 inches per.

That means the depth of snow will be:

`S(x)=1.5x\ \ \ 0\leq x< 4`

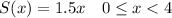
At 4:00 a.m., it starting falling at a constant rate of 3 inches per hour,

`S(x)=3(x-4)+6\ \ \ 4\leq x<7`

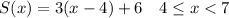
7:00 a.m. to 9:00 a.m., snow was falling at a constant rate of 2 inches per hour.

`S(x)=2(x-7)+15\ \ \ 7\leq x\leq 9`

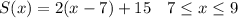
The required piece-wise linear function is

`\left\{\begin{matrix}1.5x & 0\leq x<4\\ 3(x-4)+6 & 4\leq x<7\\ 2(x-7)+15 & 7\leq x\leq 9\end{matrix}\right.`

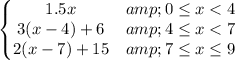
We need to find the how deep was the snow at 9:00 am

For 9:00 am we will choose the function which can take the value of x=9

Substitute the value of x=9 in
`S(x)=2(x-7)+15`

`S(x)=2(9-7)+15`

`S(x)=2(2)+15`

`S(x)=19`

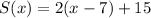
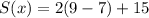
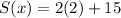
Hence, the depth of snow at 9:00 am was 19 inches.