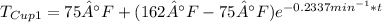Question

Suppose both cups are poured at the same time, and both are left sitting in the room that is 75°F. But this time,

milk is immediately poured into Cup 2, cooling it to an initial temperature of 162°F.
a. Use Newton’s law of cooling to write equations for the temperature of each cup of coffee after tt minutes has
elapsed.

Answer 1

The equations of both cups after t minutes is given by:

`T_(Cup1)=75 °F+(180 °F-75 °F)e^{-0.2337min^(-1)*t}`

`T_(Cup1)=75 °F+(162 °F-75 °F)e^{-0.2337min^(-1)*t}`

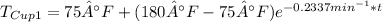
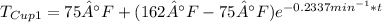
Explanation:

The complete exercise says:

Two cups of coffee are poured from the same pot. The initial temperature of the coffee is 180°F, and is 0.2337 (for time in minutes).

Newton's law of cooling:

`T=T_m+(T_0-T_m)e^(-kt)`

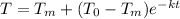
where,

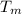`T_m`= room temperature

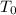`T_0`= initial temperature

k=cooling constant

Hence,

Cup 1:

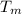`T_m`= 75 °F

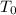`T_0`= 180 °F

`T_(Cup1)=75 °F+(180 °F-75 °F)e^{-0.2337min^(-1)*t}`

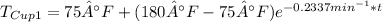
Cup 2:

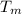`T_m`= 75 °F

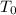`T_0`= 162 °F

`T_(Cup1)=75 °F+(162 °F-75 °F)e^{-0.2337min^(-1)*t}`