Question

In a study of government financial aid for college​ students, it becomes necessary to estimate the percentage of​ full-time college students who earn a​ bachelor's degree in four years or less. Find the sample size needed to estimate that percentage. Use a 0.04 margin of error and use a confidence level of 99​%. Complete parts​ below.

​(a) Assume that nothing is known about the percentage to be estimated.
(b) Assume prior studies have shown that about 55​% of​ full-time students earn​ bachelor's degrees in four years or less.

Answer 1

a) n = 1037.

b) n = 1026.

Explanation:

In a sample with a number n of people surveyed with a probability of a success of
`\pi`, and a confidence level of
`1-\alpha`, we have the following confidence interval of proportions.

`\pi \pm z\sqrt{(\pi(1-\pi))/(n)}`

In which

z is the zscore that has a pvalue of
`1 - (\alpha)/(2)`

The margin of error is:

`M = z\sqrt{(\pi(1-\pi))/(n)}`

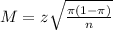
99% confidence level

So
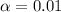`\alpha = 0.01`, z is the value of Z that has a pvalue of
`1 - (0.01)/(2) = 0.995`, so
`Z = 2.575`.

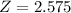​(a) Assume that nothing is known about the percentage to be estimated.

We need to find n when M = 0.04.

We dont know the percentage to be estimated, so we use
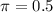`\pi = 0.5`, which is when we are going to need the largest sample size.

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.04 = 2.575\sqrt{(0.5*0.5)/(n)}`

`0.04√(n) = 2.575*0.5`

`(√(n)) = (2.575*0.5)/(0.04)`

`(√(n))^(2) = ((2.575*0.5)/(0.04))^(2)`

`n = 1036.03`

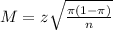
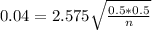
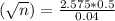
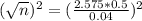
Rounding up

n = 1037.

(b) Assume prior studies have shown that about 55​% of​ full-time students earn​ bachelor's degrees in four years or less.

`\pi = 0.55`

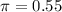
So

`M = z\sqrt{(\pi(1-\pi))/(n)}`

`0.04 = 2.575\sqrt{(0.55*0.45)/(n)}`

`0.04√(n) = 2.575*√(0.55*0.45)`

`(√(n)) = (2.575*√(0.55*0.45))/(0.04)`

`(√(n))^(2) = ((2.575*√(0.55*0.45))/(0.04))^(2)`

`n = 1025.7`

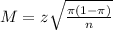
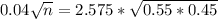
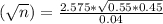
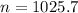
Rounding up

n = 1026.