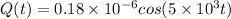Answer:
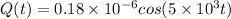
Step-by-step explanation:
We knwo that Kirchoff law

where
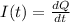
hence
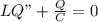
C is given as 0.04\times 10^{6} F
L= 1 H , so we have
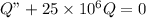
the characteristic equation of this differential equation is

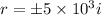
Therefore differential equation is

we know initial value if capacitor is given as

Therefore
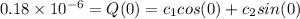
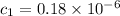
if no inital current is present then we hvae I(0) = Q'(0) = 0

 therefre
therefre
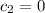
hence charge is