Question

An Atwood machine consists of two masses hanging from the ends of a rope that passes over a pulley. Assume that the rope and pulley are massless and that there is no friction in the pulley. If the masses have the values m1=20.5 kg m 1=20.5 kg and m2=11.1 kg,m2=11.1 kg, find the magnitude of their acceleration ????a and the tension TT in the rope. Use ????=9.81 m/s2.

Answer 1

a= 2.92 m/s²

T = 141.19 N

Step-by-step explanation:

Known data

m₁=20.5 kg

m₂=11.1 kg

g=9.81 m/s² acceleration due to gravity

Weigt (W) calculations

W₁ = m₁*g = 20.5 kg* 9.81 m/s² = 201.105 N

W₂ = m₂*g = 11.1 kg* 9.81 m/s² = 108.78 N

Newton's second law:

∑F = m*a (Formula 1)

∑F : algebraic sum of the forces in Newton (N)

m : mass in kilograms (kg)

a : acceleration in meters over second square (m/s²)

Problem development

We identify a direction as positive and observe that m₁ will accelerate downwards and m₂ will accelerate upwards, since m₁> m₂.

We choose the positive direction down and we apply the equations 1 :

m₁ Newton's second law

∑F = m₁*a

W₁-T = m₁*a

201.105-T =20.5*a Equation (1)

m₂ Newton's second law

∑F = m₁*a

W₂-T = m₂*a

108.78-T = 11.1*a Equation (2)

We have a system of 2 equations with two unknowns

201.105-T =20.5*a Equation (1)

T - 108.78 = 11.1*a Equation (2)

We clear T from both equations

(1) T = 201.105 - 20.5*a

(2) T = 108.78 + 11.1*a

(1) = (2)

201.105 - 20.5*a = 108.78 + 11.1*a

201.105 - 108.78 = 11.1*a + 20.5*a

92.325 = 31.6 a

`a= (92.325)/(31.6)`

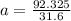
a= 2.92 m/s²

We replace a= 2.92 m/s² in (2)

T = 108.78 + 11.1*2.92

T = 141.19 N