Question

Suppose the correlation coefficient describing how the height of teenage boys predicts their weights is 0.85. What is the coefficient of determination, ???? 2 , and what does it mean in context? A ???? 2 is 0.7225. 72.25% of the variation in weights of teenage boys can be explained by their height. B) ???? 2 is 0.7225. 72.25% of the variation in heights of teenage boys can be explained by their weight. C) ???? 2 is 0.85. 85% of the variation in heights of teenage boys can be explained by their weight. D) ???? 2 is 0.85. 85% of the variation in weights of teenage boy

Answer 1

Option A) 72.25% of the variation in weights of teenage boys can be explained by their height.

Explanation:

Coefficient of determination:

• The coefficient of determination is the square of the correlation between predicted y scores and actual y scores.

• The coefficient of determination is also equal to the square of the correlation between x and y scores.

• If coefficient of determination is zero that means the dependent variable cannot be predicted from the independent variable.

• If the coefficient of determination is one the dependent variable can be predicted without error from the independent variable.

We are given that correlation coefficient of height of teenager and their weights is 0.85.

Option A) is the correct interpretation of coefficient of determination.

`\text{Coefficient of Determination}=0.85^2 = 0.7225 = 72.25\%`

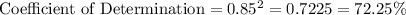
72.25% of the variation in weights of teenage boys can be explained by their height.