Question

A chemist has 200 mL of a 10% sucrose solution. She adds x mL of a 40% sucrose solution. The percent concentration, y, of the final mixture is given by the rational function:

y=0.1(200)+0.4x/200+x * 100

The chemist needs the concentration of the final mixture to be 30%. How many milliliters of the 40% solution should she add to the 10% solution to get this concentration?
mL

Answer 1

The answer is 400 ML

The 2nd answer is 600 ML

3rd answer is 180g

Answer 2

The chemist needs to add 400mL of 40% solution.

Explanation:

The equation

`y=(0.1(200)+0.4x)/(200+x) *100`

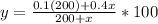
gives the percent concentration
`y` of the final mixture, when
`x` mL of the 40% solution are added.

Now we are asked, how many milliliters
`x` of the 40% solution should the chemist add to get final percent concentration
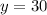`y=30`; this is just a matter of solving the equation

`30=(0.1(200)+0.4x)/(200+x) *100`

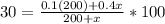
and we solve it the following way:

`30=(0.1(200)+0.4x)/(200+x) *100\\\\0.3 =(0.1(200)+0.4x)/(200+x)\\\\0.3(200+x)=0.1(200)+0.4x\\\\60+0.3x=20+0.4x\\\\40=0.1x\\\\x=(40)/(0.1) \\\\\boxed{x=400\:mL}`

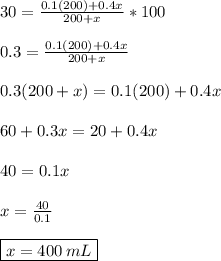
Thus, the chemist needs 400mL of 40% solution to get 30% concentration of the final mixture.