Question

If 2, a, b, −54 forms a geometric sequence, find the values of a and b.

Answer 1

The values are a=-6 and b=18.

Explanation:

A Geometric sequence is given by the formula
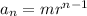`a_n=mr^(n-1)` for all
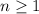`n\geq 1`, where r ≠ 0 is the common ratio and
`m` is the first term of the sequence .

In this problem we know that m= 2,
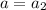`a=a_(2)` is the second term of the sequence and
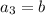`a_(3) =b` is the third term.

First we need to find the general form of the sequence, we can use the fourth term
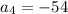`a_(4)=-54` and the value of
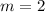`m=2` to find r.

We replace
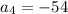`a_(4)=-54` and
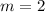`m=2` in the formula
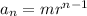`a_n=mr^(n-1)`, then we have

`a_4=mr^(4-1)`

`-54=2r^(4-1)`

`(-54)/(2) =r^3`

`-27=r^3`

`r=-3`.

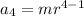
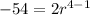
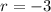Therefore the general form of the sequence is
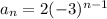`a_n=2(-3)^(n-1)` for all
`n \geq 1`.

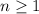To find the value of a, we replace n=2 in our formula, so

`a=a_2=2(-3)^(2-1)=2(-3)=-6`.

To find the value of b, we replace n=3 in our formula, so

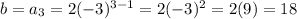`b=a_3=2(-3)^(3-1)=2(-3)^(2) =2(9)=18`.