Question

Romeo (81.0 kg) entertains Juliet (53.0 kg) by playing his guitar from the rear of their boat at rest in still water, 2.70 m away from Juliet, who is in the front of the boat. After the serenade, Juliet carefully moves to the rear of the boat (away from shore) to plant a kiss on Romeo's cheek. How far does the 79.0-kg boat move toward the shore it is facing?

Answer 1

0.64 m

Step-by-step explanation:

The first thing is calculate the center of mass of the system.

`X_(cm)= \sum_(n=1)^(n)(X_n* M_n)/(M_n)`

now multiplying every coordinate x by the mass of each object (romeo, juliet and the boat) and dividing all by the total mass taking by reference the position of juliet.

`X_(cm)=(53*0 +81*2.7+79*1.35)/(53+81+79)`

X_cm = 1.4589 m

When the forces involved are internals, the center of mass don't change

After the movement the center of mass remains in the same distance from the shore, but change relative to the rear of the boat.

`X_(cm)=(79*1.35+(81+53)*2.7)/(53+81+79)`

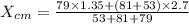
X_cm= 2.10 m

this displacement is how the boat move toward the shore.

2.10-1.46= 0.64 m