Answer:
There is a 22.66% probability that the amount dispensed into a box is less than 12 ounces.
Explanation:
Problems of normally distributed samples can be solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by
, the zscore of a measure X is given by

After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X.
In this problem:
The amount of cereal dispensed into "12-ounce" boxes of Captain Crisp cereal is normally distributed with mean 12.09 ounces and standard deviation 0.12 ounces, so
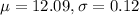 .
.
That is, what is the probability that the amount dispensed into a box is less than 12 ounces?
This is the pvalue of Z when
 .
.
So:

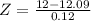

 has a pvalue of 0.2266.
has a pvalue of 0.2266.
This means that there is a 22.66% probability that the amount dispensed into a box is less than 12 ounces.