Answer:

Explanation:
A new corridor of the arena lies on the line
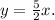
The slope of this new corridor is
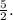
If
 is the slope of the prependicular line, then
is the slope of the prependicular line, then
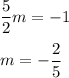
Therefore, the perpendicularcorridor has the equation

This corridor passes through the point (10,0), then
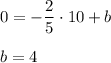
The equation of the corridor is
