Answer:
N > mg
Step-by-step explanation:
When we are at the bottom of the circle, there are two forces acting on the passenger:
- The normal force, N, upward
- The weight of the passenger (+ the cabin), mg, downward
So, the equation of the forces is
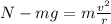
where
 is the centripetal force, which points towards the centre of the wheel, so upward (as the normal force)
is the centripetal force, which points towards the centre of the wheel, so upward (as the normal force)
m is the mass
v is the speed
r is the radius of the wheel
Re-arranging the equation,
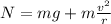
We can notice that the term
 is always positive, therefore we have
is always positive, therefore we have
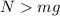
So, the normal force is larger than the weight.