Question

Lithium acetate, LiCH3CO2, is a salt formed from the neutralization of the weak acid acetic acid, CH3CO2H, with the strong base lithium hydroxide. Given that the value of Ka for acetic acid is 1.8×10−5, what is the pH of a 0.289 M solution of lithium acetate at 25∘C?

Answer 1

Answer : The pH of 0.289 M solution of lithium acetate at
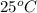`25^oC` is 9.1

Explanation :

First we have to calculate the value of
`K_b`.

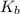As we know that,

`K_a* K_b=K_w`

where,

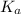`K_a` = dissociation constant of an acid =
`1.8* 10^(-5)`

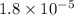
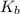`K_b` = dissociation constant of a base = ?

`K_w` = dissociation constant of water =
`1* 10^(-14)`

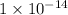
Now put all the given values in the above expression, we get the dissociation constant of a base.

`1.8* 10^(-5)* K_b=1* 10^(-14)`

`K_b=5.5* 10^(-10)`

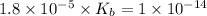
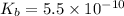
Now we have to calculate the concentration of hydroxide ion.

Formula used :

`[OH^-]=(K_b* C)^{(1)/(2)}`

where,

C is the concentration of solution.

Now put all the given values in this formula, we get:

`[OH^-]=(5.5* 10^(-10)* 0.289)^{(1)/(2)}`

`[OH^-]=1.3* 10^(-5)M`

Now we have to calculate the pOH.

`pOH=-\log [OH^-]`

`pOH=-\log (1.3* 10^(-5))`

`pOH=4.9`

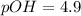
Now we have to calculate the pH.

`pH+pOH=14\\\\pH=14-pOH\\\\pH=14-4.9=9.1`

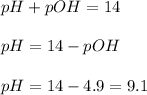
Therefore, the pH of 0.289 M solution of lithium acetate at
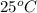`25^oC` is 9.1