Question

A state university recently implemented an experimental program for statistics education. After one year, a random sample of 36 statistics students were a part of this special program and obtained an average score of 64.8 on a national statistics achievement test. The national average for this test is (µ = 60) and a standard deviation (σ = 12). Did the students in this special program score significantly above the national average? (α = .05). 1. Appropriate test to use: _________________________________ (1 points) 2. Did the students score significantly above the national average? Explain your answer. (1 points) 3. Write the results of this test in the appropriate format: ____________________________________ (2 points) 4. What is the probability of a Type 1 Error? ______________________________________________ (1 points) 5. Explain what Type 1 Error means: ____________________________________________________ (1 points)

Answer 1

1- A critical value right-tailed Normality test.

2- Yes, they did.

3-

Null hypothesis

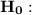`\bf H_0:` The national average on the statistics achievement test is 60

Alternative hypothesis

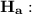`\bf H_a:` The average on the experimental program for statistics education is greater than 60

4- 0.05 or 5%

5- A Type 1 Error is the error we make when we reject the null hypothesis given that it is true.

Explanation:

1)

Since the sample size is greater than 30, the population follows a Normal distribution, and we suspect the average is greater than the established one, the appropriate test to use is a critical value right-tailed Normality test.

2)

To check if the students scored significantly above the national average in this special program to a significance level α = 0.05, we must check if the z-statistic given by the sample falls to the right of 1.64, since the area under the Normal N(0;1) to the right of 1.64 equals 0.05

Our z-statistic is given by the formula

`\bf z=(\bar x - \mu)/(\sigma/√(n))`

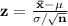
where

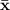`\bf \bar x` is the mean of the sample

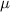`\bf \mu` is the mean of the null hypothesis

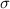`\bf \sigma` is the standard deviation

n is the sample size

Our z-statistic is then

`z=(64.8-60)/(12/√(36))=2.4`

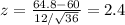
Since 2.4 > 1.64, the students did score significantly above the national average in this special program to a significance level α = 0.05

3)

Null hypothesis

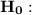`\bf H_0:` The national average on the statistics achievement test is 60

Alternative hypothesis

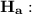`\bf H_a:` The average on the experimental program for statistics education is greater than 60

4)

The probability of a Type 1 Error is 0.05 or 5% (the significance level)

5)

A Type 1 Error is the error we make when we reject the null hypothesis given that it is true.