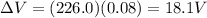Answer:
18.1 V
Step-by-step explanation:
The electric field between two parallel plates is given by the equation:
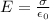
where
 is the charge surface density
is the charge surface density
 is the vacuum permittivity
is the vacuum permittivity
For the plates in this problem,
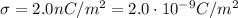
So, the magnitude of the electric field is
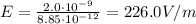
Now we can find the potential difference between the plates, which is given by
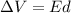
where
d = 8.0 cm = 0.08 m is the separation between the plates
Substituting,