Question

In a particular roulette game, there's a 1/36 chance of winning. In a single day, a gambler plays 100 rounds, and wins in 7 of them. What's the P-value for winning 7 or more out of 100 rounds? (Hint: Be sure you know which kind of probability distribution you're dealing with before you do the calculation.)

Answer 1

0.021739

Explanation:

This situation can be modeled with the Binomial Distribution which gives the probability of an event that occurs exactly k times out of n, and is given by

`\large P(k;n)=\binom{n}{k}p^kq^(n-k)`

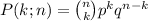
where

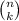`\large \binom{n}{k}`= combination of n elements taken k at a time.

p = probability that the event (“success”) occurs once

q = 1-p

In this case, the event “success” is winning the roulette game with probability 1/36 = 0.027777 and n=100 rounds.

The probability value of winning the roulette game 7 or more times out of 100, is

P(7;100)+P(8;100)+...+P(100;100) =

1 - P(0;100)+P(1;100)+...+P(6;100)

We can find the sum of these last 7 terms either by hand or computer-assisted and we would find

P(0;100)+P(1;100)+...+P(6;100) =

`\large \binom{100}{0}0.027777^0*0.972222^(100)+\binom{100}{1}0.027777^1*0.972222^(99)+...+\\\binom{100}{100}0.027777^(100)*0.972222^0=0.978261`

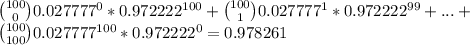
and the p-value (probability value) for winning 7 or more out of 100 rounds is

1-0.978261 = 0.021739