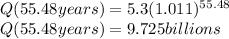Answer:
a)55.48 years
b)9.725 billions
Explanation:
First of all, note that when you increase certain amount by x percent, you only have to multiply that amount for a decimal number following this rule:
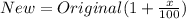
For example, if you increase 5.3 billion by 20%, then:
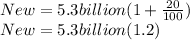
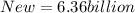
In the problem you need to increase the population by 2%/year, then after one year you'll have:
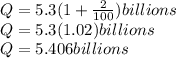
Note that this last quantity will increase 2% in the second year, then:
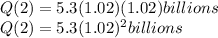
In the third year the population will be:

Then, the function Q(t) that expresses the world population (in billions) is given by:

where t=0 corresponds to the beginning of 1990 (5.3 billions).
a)The time necessary for the population to triple in size is given by:
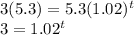
To solve for t, you need to apply the natural logarithm or the common logarithm in both sides of the equation:
![ln(3)=ln[(1.02)^(t)]\\ln(3)=t(ln(1.02))\\t=(ln(3))/(ln(1.02))\\ t=55.48 years](https://img.qammunity.org/2020/formulas/mathematics/high-school/srs9a7hzc1l4mk9hdp7vr9490doxwco0j3.png)
Then, the time required to the population to triple in size is 55.48 years.
b)If the growth rate were reduced to 1.1%/year, the function would be:
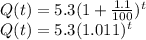
The world population at the time obtained in a) would be: