Answer:
Divide both sides of the inequality by -2:

Explanation:
The Division property of Inequality states that:
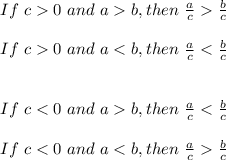
Therefore, given the inequality:

The missing step in solving it, applying the Division property of inequality, is divide both sides of the inequality by -2:
