Question

At elevated temperatures, molecular hydrogen and molecular bromine react to partially form hydrogen bromide:

H2 (g) + Br2 (g) <-> 2HBr (g)

A mixture of 0.682 mol of H2 and 0.440 mol of Br2 is combined in a reaction vessel with a volume of 2.00 L. At equilibrium at 700 K, there are 0.566 mol of H2 present. At equilibrium, there are _______ mol of Br2 present in the reaction vessel.

Answer 1

Answer: The moles of bromine gas at equilibrium is 0.324 moles.

Step-by-step explanation:

To calculate the molarity of solution, we use the equation:

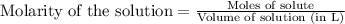`\text{Molarity of the solution}=\frac{\text{Moles of solute}}{\text{Volume of solution (in L)}}` .......(1)

Calculating the initial moles of hydrogen and bromine gas:

• For hydrogen gas:

Moles of hydrogen gas = 0.682 mol

Volume of solution = 2.00 L

Putting values in equation 1, we get:

`\text{Molarity of solution}=(0.682mol)/(2.00L)=0.341M`

• For bromine gas:

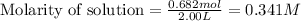
Moles of bromine gas = 0.440 mol

Volume of solution = 2.00 L

Putting values in equation 1, we get:

`\text{Molarity of solution}=(0.440mol)/(2.00L)=0.220M`

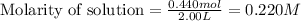
Now, calculating the molarity of hydrogen gas at equilibrium by using equation 1:

Equilibrium moles of hydrogen gas = 0.566 mol

Volume of solution = 2.00 L

Putting values in equation 1, we get:

`\text{Molarity of solution}=(0.566mol)/(2.00L)=0.283M`

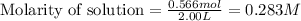
Change in concentration of hydrogen gas = 0.341 - 0.283 = 0.058 M

This change will be same for bromine gas.

Equilibrium concentration of bromine gas =
`(\text{Initial concentration}-\text{Change in concentration})=0.220-0.058=0.162M`

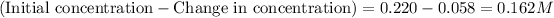
Now, calculating the moles of bromine gas at equilibrium by using equation 1:

Molarity of bromine gas = 0.162 M

Volume of solution = 2.00 L

Putting values in equation 1, we get:

`0.162M=\frac{\text{Moles of bromine gas}}{2.00L}\\\\\text{Moles of bromine gas}=(0.162mol/L* 2.00L)=0.324mol`

Hence, the moles of bromine gas at equilibrium is 0.324 moles.