Question

Suppose that 25% of all students who have to buy a physical copy of the 9th Edition of our textbook want a new copy (the "successes"), whereas the other 75% want a used copy. Each chooses independently of the others. Consider randomly selecting 30 purchasers. a) What is the expected value of the number of students who want a new copy of the book? Please write out all formulas in your work. Answer:

Answer 1

7 or 8

Explanation:

This situation can be modeled with the Binomial Distribution which give the probability of an event that occurs exactly k times out of n, and is given by

`\large P(k;n)=\binom{n}{k}p^kq^(n-k)`

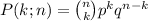
where

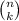`\large \binom{n}{k}`= combination of n elements taken k at a time.

p = probability that the event (“success”) occurs once

q = 1-p

In this case, the event “success” is wanting a new copy of the book with probability 25% = 0.25 and n=30 students randomly chosen.

The expected value for a binomial distribution is the mean np, so in this case the expected value of the number of students who want a new copy of the book would be

np = 30*0.25 = 7.5

To make sense of this value, we could say that 7 or 8 students out of 30 want a new copy of the book.