Question

A helicopter is lifting two crates simultaneously. One crate with a mass of 2.800 × 102 kg is attached to the helicopter by a cable. The second crate with a mass of 1.700 × 102 kg is hanging below the first crate and attached to the first crate by a cable. As the helicopter accelerates upward at a rate of 1.39 m/s2, what is the tension in each of the two cables?

Answer 1

Step-by-step explanation:

It is given that,

Mass of crate 1,
`m_1=2.8* 10^2\ kg`

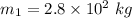
Mass of the crate 2,
`m_2=1.7* 10^2\ kg`

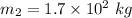
It is also mentioned that the second crate is hanging below the first crate and attached to the first crate by a cable.

The acceleration of the helicopter,
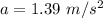`a=1.39\ m/s^2` (upwards)

The tension in the cable 1 is given by :

`T_1=m_1(a+g)`

`T_1=2.8* 10^2(1.39+9.8)`

`T_1=3133.2\ N`

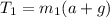
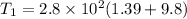
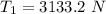
The tension in the cable 2 is given by :

`T_2=(m_1+m_2)(a+g)`

`T_2=(2.8* 10^2+1.7* 10^2)* (1.39+9.8)`

`T_2=5035.5\ N`

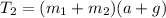
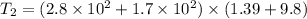
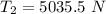
Hence, this is the required solution.