Question

Is it possible to ionize an atom of 133Cs, initially at rest, by a collision with an atom of 16O that has kinetic energy Kinitial of 4.0 electron volts? The ionization energy of the cesium atom is 3.9 electron volts. It doesn't matter what energy units you choose, as long as you are consistent. For this question, it is most convenient to use electron volts (eV) throughout. Note that 1 eV=1.60×10−19 J, which you maybe more familiar with.

Answer 1

ΔK = 3.57 eV We can see this is less than the energy needed to ionize the atom so the process does not occur

Step-by-step explanation:

In order to respond we must know what the energy of the atoms is after the shock. We create a system formed by the two atoms, whereby the amount of movement is conserved

p₀ = m v₀

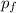`p_(f)` = (m + M) v

p₀ =
`p_(f)`

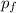
v = m / (m + M) v₀

Let's look for the initial velocity of the oxygen atom

K = ½ m v₀²

v₀ = √2K / m

We calculate the final system speed

v = m / (m + M) (√ 2K/m)

We found the final energy

Kf = ½ (m + M) v2

Kf = ½ (m + M) [m² / (m + M)² 2K/m]

Kf = m/(m + M) K

Let's replace and calculate

Kf = 16u / (16u + 133u) K

u = 1.67 10⁻²⁷ kg

Kf = 16/149 K

Kf = 0.107 K

Kf = 0.107 4.0

Kf = 0.428 eV

This is the kinetic energy of the system after the collision, what remains is the energy available for ionization

ΔK = Ko -Kf

ΔK = 4 - 0.428

ΔK = 3.57 eV

We can see this is less than the energy needed to ionize the atom so the process does not occur