Question

Rectarigle is six meters less than its length

the area of the rectangle is 112 m², find
**TUIVU:
length. If the area of the rect:
the dimensions of the rectanal

Answer 1

Answer:23

Step-by-step explanation:yes

Answer 2

Length = 14 meters

Width = 8 meters

Explanation:

Let x meters be the length of the rectangle. Rectangle's width is 6 meters less than its length, then the width of the rectangle is x - 6 meters.

Find the area of the rectangle:

`A=x(x-6)\\ \\112=x(x-6)\\ \\x^2-6x=112\\ \\x^2-6x-112=0`

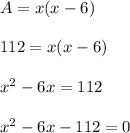
Solve this equation:

`D=b^2-4ac=(-6)^2-4\cdot 1\cdot (-112)=36+448=484\\ \\x_(1,2)=(-b\pm √(D))/(2a)=(-(-6)\pm 22)/(2)=(6\pm 22)/(2)=-8,\ 14`

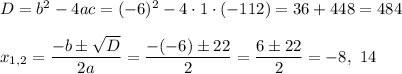
Thw length cannot be negative, so x = 14 meters, then x - 6 = 8 meters is the width.