Answer: 0.21
Explanation:
Let A denotes the event that employees are college graduates.
B denotes that the event that employees have more than ten years of experience.
As per given , we have
P(A)=0.51
P(B)=0.47
P(A∪B)=0.77
We know that ,
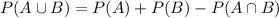
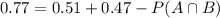
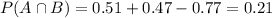
Hence, the probability that a randomly selected employee will have more than ten years of experience and be a college graduate = 0.21