Question

A satellite is put into an orbit at a distance from the center of the Earth equal to twice the distance from the center of the Earth to the surface. If the satellite had a weight at the surface of 4000 N, what is the force of gravity (weight) of the satellite when it is in its orbit? Give your answer in newtons, N.

Answer 1

995 N

Step-by-step explanation:

Weight of surface, w= 4000N

Gravitational constant, g, is taken as 9.81 hence mass, m of surface is W/g where W is weight of surface

m= 4000/9.81= 407.7472

Using radius of orbit of 6371km

The force of gravity of satellite in its orbit,
`F=\frac {GMm}{(2r)^(2)}=\frac {GMm}{4(r)^(2)}`

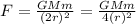
Where
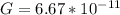`G=6.67*10^(-11)` and
`M=5.94*10^(24)`

`F=\frac {(6.67*10^(-11)*5.94*10^(24)*407.7472)}{4*({6.371*10^(6)m)}^(2)}`

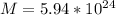
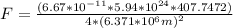
F= 995.01142 then rounded off

F=995N