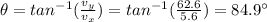a) 5.6 m/s
First of all, we consider the vertical motion of the stone, which is a free fall motion (uniform accelerated motion). We can use the suvat equation :
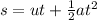
where
s = 200 m is the vertical displacement (here we chose downward as positive direction)
u = 0 is the initial vertical velocity of the stone (since it is launched horizontally)
t is the time
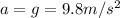 is the acceleration of gravity
is the acceleration of gravity
Solving for t, we find the time the stone takes to reach the ground:
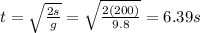
Now we can consider the horizontal motion: this is a uniform motion with constant speed. The horizontal distance travelled is given by
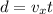
where
d = 36.0 m is the horizontal distance travelled by the stone
t = 6.39 s is the time of flight
If we solve for
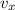 , we find the speed at which the stone was launched (which remains constant during the whole motion):
, we find the speed at which the stone was launched (which remains constant during the whole motion):

(b) 62.8 m/s at
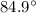 below the horizontal
below the horizontal
- The motion along the horizontal direction is a uniform motion, since there are no forces acting on the stone in this direction - so the horizontal velocity remains constant:
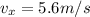
- The vertical velocity instead changes due to the effect of the acceleration of gravity. We can calculate the vertical velocity at the time of impact by using the equation
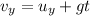
where
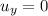 is the initial vertical velocity (zero because the stone is launched horizontally)
is the initial vertical velocity (zero because the stone is launched horizontally)
Solving for t = 6.39 s, we find:
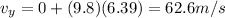
Keep in mind that the direction of this velocity is downward.
So now we can find the speed of the stone at the moment of impact:

And the angle of impact, measured as below the horizontal (since the vertical velocity is downward) is