Question

Which is the best estimate for the average rate of change for the quadratic function graph on the interval −3 ≤ x ≤ −2?

Answer 1

-4

Explanation:

Answer 2

-5a+b

Explanation:

A general quadratic function of x is given by f(x) = ax² +bx +c

Now, the rate of change of the quadratic function f(x) is given by
`(df(x))/(dx)`.

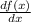Therefore,
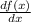`(df(x))/(dx)` = 2ax +b.

Now, the value of x ranges between -3 ≤ x ≤-2.

So, at x= -3,
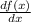`(df(x))/(dx)` = 2a(-3) +b = -6a +b

And at x= -2,
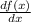`(df(x))/(dx)` = 2a(-2) +b = -4a +b

Therefore, the average rate of change will be given by
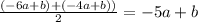`((-6a+b)+(-4a+b)))/(2) =-5a+b`. (Answer)