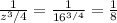Question

84. Use properties of exponents to rewrite each expression with only positive, rational exponents. Then find the numerical value of each expression when x = 9, y= 8, and z= 16. In each case, the expression evaluates to a rational number.

C. X-3/2 Y4/3 Z-3/4

Answer 1

a) 1/27

b) 16

c) 1/8

Explanation:

a)
`x^(-3/2)`

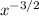
One of the properties of the exponents tells us that when we have a negative exponent we can express it in terms of its positive exponent by turning it into the denominator (and changing its sign), so we would have:

`x^(-3/2)=(1)/(x^(3/2) )`

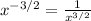
And now, solving for x = 9 we have:

`(1)/(x^(3/2))=(1)/(9^(3/2) )  =(1)/(27)`

b)
`y^(4/3)`

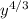
This is already a positive rational exponent so we are just going to substitute the value of y = 8 into the expression

`y^(4/3)=8^(4/3)=16`

c)
`z^(-3/4)`

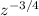
Using the same property we used in a) we have:

`z^(-3/4)=(1)/(z^(3/4) )`

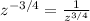
And now, solving for z = 16 we have:

`(1)/(z^3/4) } =(1)/(16^(3/4) ) =(1)/(8)`