Question

Ahmed learned: "To multiply a whole number by ten, just place a zero at the end of the number." For

example, 2813 × 10, he says, is 28,130. He doesn't understand why this rule is true.
a. What is the product of the polynomial 2x³ + 8x² + x + 3 times the polynomial x?
b. Use part (a) as a hint. Explain why the rule Ahmed learned is true.

Answer 1

a) 2x^4 + 8x^3 + x^2 + 3x

b) evaluate this polynomial at x = 10

Explanation:

Hi!

a)

The product of a sum is the sum of the products, having said this:

`(2x^3 + 8x^2 + x + 3) * x = 2x^4 + 8x^3 + x^2 + 3x`

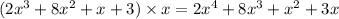
b)

We can use the result from part a and consider x = 10.

First lets take the first polynomial

`2x^3 + 8x^2 + x + 3 = 2(10)^3 + 8 (10)^2+10^1+3(10)^0`

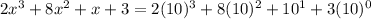
Knowing that every number (excepting probably 0, which could be undefined for some or 1 for others) to the power of 0 is one:

`2x^3 + 8x^2 + x + 3 = 2(1000)+ 8(100)+10+3(1) = 2813`

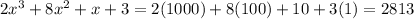
Which is the initial number

Now lets conisder the product of the polynomial times x and evaluate it at x=10:

`(2x^3 + 8x^2 + x + 3) * x = 2x^4 + 8x^3 + x^2 + 3x`

`(2(10)^4 + 8(10)^3 + 10^2 + 3(10) = 2 (10000) + 8(1000) + 100 + 3(10)`

`= 28130`

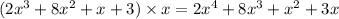
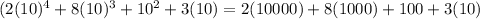
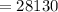
We can now see that teh rule is treu because we can see the whole number as a sum of numbers from 0 to 9 multiplied by a power of 10, and when it is multiplied by 10, each power increases by one, and at the end, the final result is adding a zero at the end