Given that,
velocity, v = 7.8 ft/s
Radius, r = 1.25 ft
To find,
a. What is the acceleration of the bucket?
b. What is the velocity if the acceleration is 25 ft/sec²?
Solution,
(a) The centripetal acceleration is given by :
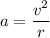
Put v = 7.8 ft/s and r = 1.25 ft
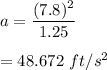
(b) Put a = 25 ft/s² to find velocity.
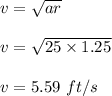
Hence, this is the required solution.