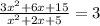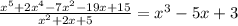Answer:
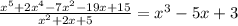
Explanation:
To find the quotient we need to apply long division on
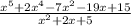 , as follows:
, as follows:
- Divide
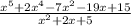
Divide the leading coefficients of the numerator
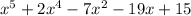 and the divisor
and the divisor
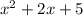
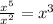
Quotient =
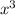
Multiply
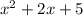 by
by
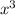 =
=
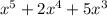
Subtract
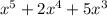 from
from
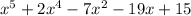 to get new remainder
to get new remainder
Remainder =

Therefore
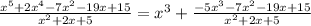
2. Divide
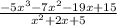
Divide the leading coefficients of the numerator
 and the divisor
and the divisor
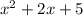
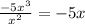
Quotient =
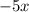
Multiply
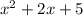 by
by
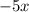 =
=
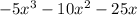
Subtract
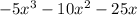 from
from
 to get new remainder
to get new remainder
Remainder =
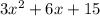
Therefore

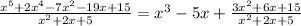
3. Divide
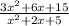
Divide the leading coefficients of the numerator
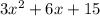 and the divisor
and the divisor
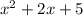

Quotient = 3
Multiply
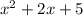 by 3 =
by 3 =
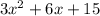
Subtract
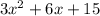 from
from
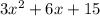 to get new remainder
to get new remainder
Remainder = 0
Therefore