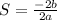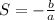Question

Find a formula for the sum S of the solutions of the quadratic equation ax²+bx+c=0 .

Answer 1

`S = -(b)/(a)`

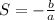
Explanation:

Given a second order polynomial expressed by the following equation:

`ax^(2) + bx + c, a\\eq0`

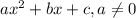
This polynomial has roots
`x_(1), x_(2)` such that
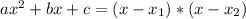`ax^(2) + bx + c = (x - x_(1))*(x - x_(2))`, given by the following formulas:

`x_(1) = (-b + √(\bigtriangleup))/(2*a)`

`x_(2) = (-b - √(\bigtriangleup))/(2*a)`

`\bigtriangleup = b^(2) - 4ac`

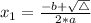
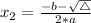
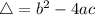
In this problem, the sum S of the solutions of a quadratic equation is:

`S = x_(1) + x_(2)`

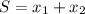
So

`S = (-b + √(\bigtriangleup))/(2*a) + (-b - √(\bigtriangleup))/(2*a)`

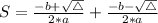
They have the same denominators, so we can keep the denominators and sum the numerators.

`S = (-b + √(\bigtriangleup) - b - √(\bigtriangleup))/(2a)`

`S = (-2b)/(2a)`

`S = -(b)/(a)`