Answer:
3.2 is the highest value for k that will yield a non-negative discriminant
Explanation:
For an equation to have real numbers as solutions, its discriminant (delta value) must be bigger than zero since there are no real square root solutions for negative numbers.
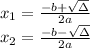
In this case, the discriminant is given by:
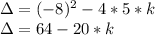
For k =3.2

As shown above, 3.2 is the highest value for k that will yield a non-negative discriminant and, therefore, if k > 3.2 the solutions of the expression are not real numbers.