Question

Solve forx.
log(x) = log(y) + log(z) + log(y) − log(z)

Answer 1

x = y²

Explanation:

Given:

log(x) = log(y) + log(z) + log(y) − log(z) ................(1)

Now,

from the properties of natural log, we have

log(A) + log(B) = log(AB)

and

log(A) - log(B) =
`\log((A)/(B))`

applying the above property in the provided equation, we have

log(x) = ( log(y) + log(z) ) + log(y) − log(z)

or

log(x) = log(yz) + log(y) - log(z)

or

log(x) = log(yzy) - log(z) [as log(yz) + log(y) = log(yzy) ]

or

log(x) = log(y²z) - log(z)

also,

log(x) =
`\log((y^2z)/(z))`

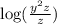
or

log(x) = log(y²)

Now, taking the anti-log both sides, we get

x = y²