Answer:
x = 16
Explanation:
Given:
log₂(x) = 4
Now,
From the properties of log
logₓ (z)=
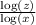 (where the base of the log is equal for both numerator and the denominator)
(where the base of the log is equal for both numerator and the denominator)
also,
log(xⁿ) = n × log(x)
thus,
using the above properties, we can deduce the results as:
logₓ(y) = n is equivalent to y = xⁿ
therefore,
the given equation can be deduced as:
log₂(x) = 4
into,
x = 4²
or
x = 16