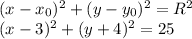Question

Write the general equation for the circle that passes through the points: (0,0) (6,0) (0,-8)

Answer 1

`(x-3)^2+(y+4)^2=25`

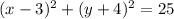
Explanation:

We start by writing the general form of the equation of a circle of radius R centered at
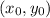`(x_0,y_0)`and creating three equations (one for each unknown:
`x_0`,
`y_0`, and the radius R):

`(x-x_0)^2+(y-y_0)^2=R^2`

1) If the circle passes through (0,0) then we should have that the equation above holds true:

`(x-x_0)^2+(y-y_0)^2=R^2\\(0-x_0)^2+(y-y_0)^2=R^2\\x_0^2+y_0^2=R^2\\R^2-x_0^2-y_0^2=0`

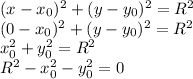
2) If the circle passes through (6,0) then we should have that the equation above holds true, and we also can use the important result from part 1) (
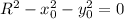`R^2-x_0^2-y_0^2=0`) to solve for
`x_0`:

`(x-x_0)^2+(y-y_0)^2=R^2\\(6-x_0)^2+(y-y_0)^2=R^2\\36-12x_0+x_0^2+y_0^2=R^2\\-12x_0+36=R^2-x_0^2-y_0^2=0\\36=12x_0\\x_0=(36)/(12) =3`

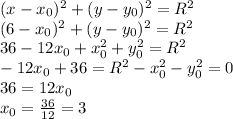
3) If the circle passes through (0,-8) then we should have that the general equation of the circle above holds true, and we also can use the important result from part 1) (
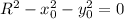`R^2-x_0^2-y_0^2=0`) to solve for
`y_0`:

`(x-x_0)^2+(y-y_0)^2=R^2\\(0-x_0)^2+(-8-y_0)^2=R^2\\x_0^2+64+16y_0+y_0^2=R^2\\64+16y_0=R^2-x_0^2-y_o^2=0\\16y_0=-64\\y_0=-(64)/(16)= -4`

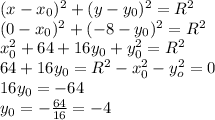
4) and finally, we use the results for
`x_0` and
`y_0` of parts 2) and 3) back into the equation in part 1) to solve for
`R^2`:

`R^2-x_0^2-y_0^2=0\\R^2-(3)^2-(-4)^2=0\\R^2-9-16=0\\\R^2=9+16=25\\`

Then, replacing
`x_0`,
`y_0`, and
`R^2` for the values we found, the equation of the circle becomes:

`(x-x_0)^2+(y-y_0)^2=R^2\\(x-3)^2+(y+4)^2=25`