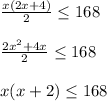Question

The height of a triangle is 4 in. Greater than twice its base. The area of the triangle is no more than 168 in.2. Which inequality can be used to find the possible lengths?

Answer 1

OPTION B:
`x(x+2)\leq 168`

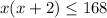
Explanation:

The complete exercise is:

"The height of a triangle is 4 in. greater than twice its base. The area of the triangle is no more than 168 in². Which inequality can be used to find the possible lengths, x, of the base of the triangle?

`A. x(x+2)\geq168\\B. x(x+2)\leq 168\\\\C.(1)/(2)x(x+4)\leq 168\\\\D. (1)/(2)x(x+4)\geq 168\\`"

The area of a triangle can be calculated with this formula:

`A=(bh)/(2)`

Where "b" is the base and "h" is the height.

Let be "x" the length of the base of the triangle and "y" the height of the triangle.

Since the area of the triangle is no more than 168 in², we can write the following inequality:

`(xy)/(2)\leq 168`

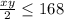
We know that the height of a triangle is 4 inches greater than twice its base, then we can write this equation:

`y=2x+4`

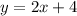
Substituting the equation into the inequality and then simplifying, we get the following inequality, which can be used to find the possible lengths of the base of the triangle:

`(x(2x+4))/(2)\leq 168\\\\(2x^2+4x)/(2)\leq 168\\\\x(x+2)\leq 168`