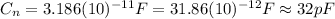Answer: 32pF
Step-by-step explanation:
The capacitance
 of a parallel plate capacitor is given by the following equation:
of a parallel plate capacitor is given by the following equation:
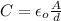
Where:
 is the electric constant when the dielectric material is vacuum
is the electric constant when the dielectric material is vacuum
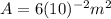 is the area of each plate
is the area of each plate
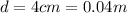 is the separation distance between both plates
is the separation distance between both plates
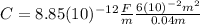
 This is the capacitance when the dielectric is vacuum.
This is the capacitance when the dielectric is vacuum.
Now, we can calculate the capacitance
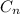 , when we have a dielectric material with a dielectric constant
, when we have a dielectric material with a dielectric constant
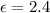 :
:
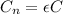

Finally: