Answer: It decreases.
Step-by-step explanation:
Lever arm is defined as the "perpendicular distance that exists from the rotation axis to the line of action of the force". Being its magnitude
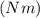 related to the application of the force that produces a torque.
related to the application of the force that produces a torque.
To understand it better:
The lever arm is an effective distance to apply a force with respect to a certain point, and this distance serves as an effective applied force amplification factor.
In this sense, Torque
 is defined as:
is defined as:
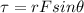
Where:
 is the distance from the applied force to the fulcrum
is the distance from the applied force to the fulcrum
 is the applied force
is the applied force
 is the angle between
is the angle between
 and
and

It is important to note,
 is maximum when
is maximum when
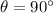 . Then, assuming
. Then, assuming
 and
and
 remains the same, if
remains the same, if
 decreases
decreases
 decreases, as well.
decreases, as well.
Hence:
If the angle
 decreases, the magnitude of the lever arm will decrease.
decreases, the magnitude of the lever arm will decrease.
So, the greater the lever arm, the greater the torque and the lesser the force we have to apply.