Question

Aiko stands 5 m from a tree. At that distance, the angle of elevation from the ground to the top of the tree is 80°.

Approximately how tall is the tree?

Answer 1

`\boxed {\boxed {\sf About \ 28.36 \ meters }}`

Explanation:

Assuming the tree is perpendicular to the ground, we can use the right triangle trigonometric ratios to find the tree's height.

• sin(θ)= opposite/hypotenuse
• cos(θ)= adjacent/hypotenuse
• tan(θ)= opposite/adjacent

Now, let's draw a diagram. We know Aiko is 5 meters from the base of the tree. From there, the angle to the top of the tree is 80 degrees. We are looking for x, the tree's height. The diagram attached is not to scale.

We base the sides off of the angle. x is opposite of 80 degrees and 5 is adjacent. Therefore we must use tangent.

`tan(\Theta)=(opposite)/(adjacent)`

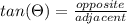
• opposite=x
• adjacent=5 m
• θ=80

Substitute in the known variables.

`tan(80)=(x)/(5 \ m )`

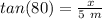
We want to find x, the height of the tree. Therefore we need to isolate that variable.

x is being divided and the inverse operation is multiplication. Multiply both sides of the equation by 5 meters.

`5 \ m* tan(80)=(x)/(5 \ m )* 5 \ m`

`5 \ m* tan(80)=x`

`5 \ m *5.67128182=x`

`28.3564091 \ m = x`

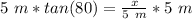
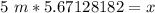
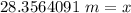
The question asks for an approximation, so let's round to the nearest hundredth.

The 6 in the thousandth place tells us to round the 5 to a 6.

`28.36 \ m \approx x`

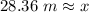
The tree is about 28.36 meters tall.