Question

a 0.04kg ball tied to a string moves in a circle that has a radius of 0.70 m. If the ball is accelerating 43.2m/s, what is the tangential velocity of the ball?​

Answer 1

Tangential Velocity = 30.24 m/s

Step-by-step explanation:

Given that,

Mass of ball, m = 0.04 Kg

Length of the string, r = 0.70 m

Acceleration of the ball, a = 43.2 m/s²

The tangential velocity of ball, V = ?

The centripetal force is given by the relation

Fc = mV²/r newton

where, m - mass of body

V - tangential velocity of body

r - radius of the trajectory

Force applied on the ball to rotate on a circular path

F = m x a newton

The applied force is equal to centripetal force.

So, equalizing the force equations

m x a = m V²/r

Therefore

V² = a x r

V =
`√(a X r)`

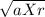
Substituting the values

V =
`√(43.2 X 0.70)`

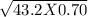
V = 30.24 m/s

So, the tangential velocity of the ball is 30.24 m/s