Answer:
The angle measurements between the diagonals are 60° and 120°
Explanation:
Let
D -----> the diagonal of rectangle
x -----> the length of rectangle
y ----> the width of rectangle
we know that
Applying the Pythagoras Theorem
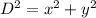 -----> equation A
-----> equation A
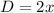 ----> equation B
----> equation B
substitute equation B in equation A
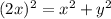
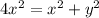
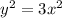
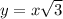
see the attached figure to better understand the problem
The triangle DOC is an isosceles triangle
m∠ODC= m∠OCD
Find the measure of angle m∠ODC
tan(∠ODC)=y/x
Remember that
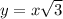
substitute
tan(m∠ODC)=x√3/x
tan(m∠ODC)=√3
m∠ODC=arc tan(√3)
m∠ODC=60°
m∠OCD=60°
Find the measure of angle m∠DOC
Remember that the sum of the internal angles of triangle must be equal to 180 degrees
The triangle DOC is an isosceles triangle
m∠ODC= m∠OCD
so
m∠ODC+ m∠OCD+m∠DOC=180°
substitute the given values
60°+ 60°+m∠DOC=180°
120°+m∠DOC=180°
m∠DOC=180°-120°=60°
Remember that the angle measurements between the diagonals are
m∠DOC and m∠BOC
The sum of the angle measurements between the diagonals is equal to 180 degrees, because are supplementary angles (form a linear pair)
we have
m∠DOC=60°
so
m∠BOC=120°
therefore
The angle measurements between the diagonals are 60° and 120°