Question

a skim jumper travels downa a slope and leaves the ski track moving in the horizontal direction with a speed of 20 m/s as in the figure. The landing incline belowe her falls off with a slope of 39degrees. the acceleration of gravity is 9.8m/s2 . calculate the distance d she travels along the incline before landing

Answer 1

85.1 m

Step-by-step explanation:

The x and y coordinates are as follows

`x=dcos\theta`

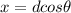
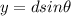`y=dsin\theta` where d is distance travelled and
`\theta` is the slope of landing

The horizontal distance travelled is
`x=vt= dcos\theta` where t is time take to travel and v is the velocity

Making t the subject,
`t=\frac {dcos\theta}{v}`

Also, the vertical distance
`y=0.5gt^(2)=dsin\theta but t= t=\frac {dcos\theta}{v}`

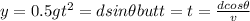
Therefore,

`dsin\theta=0.5g(\frac {dcos\theta}{v})^(2)` and making d the subject of the formula

`d=\frac {2v^(2)sin\theta}{gcos^(2)\theta}`

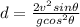
Substituting v for 20 m/s,
`\theta` for
`39*{o}` and g for
`9.81 m/s^(2)`

`d=\frac {2*(20 m/s)^(2)*sin39^(o)}{9.8 m/s ^(2)cos^(2)39^(o)}`

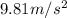
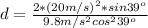
d=85.06100825

Rounding off, d=85.1 m