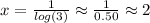Explain how to solve 3x − 4 = 6 using the change of base formula log base b of y equals log y over log b. Include the solution for x in your answer. Round your answer to the nea…

Question

asked Feb 25, 2020 143k views

1 Answer

Eftekhari · Answer 1 · 2020-03-01T23:15:28+0000

Answer:

$x \approx 2$

Explanation:

The given equation is

3^(x)-4=6

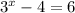
First, we move all constant terms to one side

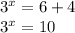
$3^(x)=6+4\\ 3^(x)=10$

Observe that the bases are different, so first, we rewrite the expression as logarithm

x=log_(3)(10)

But, we need a logarithm with base 10, so we apply the following property

log_(a)(b)=(log(b))/(log(a))

x=(log(10))/(log(3))

But,
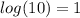
log(10)=1

So,

$x=(1)/(log(3)) \approx (1)/(0.50) \approx 2$