Question

If a 320.0 mL sample of a gas is cooled at constant pressure from 55.0ºC to 30.0ºC, its new volume is _____

mL?

Answer 1

New volume is 295.61 mL

Step-by-step explanation:

According Charles's law, at constant pressure the volume of a fixed mass of a gas and its absolute temperature are directly proportional.

Mathematically;

`V\alpha T`

`V=kT`

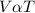
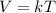
When the variables are varied then we have a constant equivalent to;

`K=(V1)/(T1) =(V2)/(T2)`

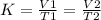
In this case, we are given;

Initial volume, V1 = 320.0 ML

Initial temperature, T1 = 55 °C or 328 K

New temperature, T2 = 30 °C or 303 K

We are required to calculate the new volume

This can be done by replacing the values of each variable in the equation;

`(V1)/(T1) =(V2)/(T2)`

we get;

`(320)/(328) =(V2)/(303)`

`V2 =((320)/(328))(303)`

`V2 = 295.609 mL`

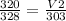
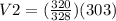
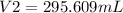
= 295.61 mL (2 d.p.)

Thus; the new volume is 295.61 mL